cot−1 Calculator
x= adjacent_side/opposite_side
cot−1(x)
Calculating Inverse Cotangent of a Value
The inverse cotangent (cot⁻¹) function, also known as arccotangent, is used to find the angle whose cotangent is a given value. This function is useful in trigonometry when you need to determine the angle based on the ratio of the adjacent side to the opposite side in a right-angled triangle.
The inverse cotangent of a value \( x \) is defined as:
\( \theta = \cot^{-1}(x) \)
where:
- θ is the angle corresponding to the cotangent value.
- x is the cotangent value, representing the ratio of the adjacent side to the opposite side.
Consider the following triangle ABC, right-angled at vertex B.
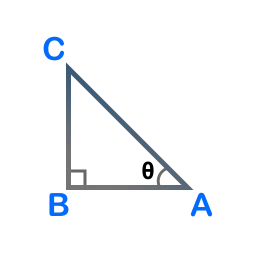
For the angle \( \theta \) at vertex A:
- Adjacent side = AB
- Opposite side = BC
Therefore,
\( \cot^{-1}(x) = \theta \) where \( x = \dfrac{\text{length of side AB}}{\text{length of side BC}} \)
Examples
The following examples demonstrate how to use the inverse cotangent function to find the angle when the cotangent value is known.
1. A surveyor is measuring the angle of depression from the top of a hill to a point on the ground 100 meters away horizontally. If the height of the hill is 50 meters, what is the angle of depression?
Answer
Given:
- Length of the adjacent side (horizontal distance) = 100 meters
- Length of the opposite side (height of the hill) = 50 meters
First, calculate the cotangent of the angle:
\( \cot(\theta) = \dfrac{\text{horizontal distance}}{\text{height of the hill}} = \dfrac{100}{50} \)
Simplify the expression:
\( \cot(\theta) = 2 \)
Now, find the angle using the inverse cotangent function:
\( \theta = \cot^{-1}(2) \)
Using a calculator or reference table, the angle is:
∴ θ ≈ 26.6°
2. A ship is anchored 150 meters offshore. The distance from the base of a cliff to the ship is 120 meters along the water. If the height of the cliff is 30 meters, what is the angle of elevation from the ship to the top of the cliff?
Answer
Given:
- Length of the adjacent side (distance along the water) = 120 meters
- Length of the opposite side (height of the cliff) = 30 meters
First, calculate the cotangent of the angle:
\( \cot(\theta) = \dfrac{\text{distance along the water}}{\text{height of the cliff}} = \dfrac{120}{30} \)
Simplify the expression:
\( \cot(\theta) = 4 \)
Now, find the angle using the inverse cotangent function:
\( \theta = \cot^{-1}(4) \)
Using a calculator or reference table, the angle is:
∴ θ ≈ 14.0°
3. A ski slope descends 60 meters over a horizontal distance of 180 meters. What is the angle of the slope with respect to the horizontal?
Answer
Given:
- Length of the adjacent side (horizontal distance) = 180 meters
- Length of the opposite side (vertical descent) = 60 meters
First, calculate the cotangent of the angle:
\( \cot(\theta) = \dfrac{\text{horizontal distance}}{\text{vertical descent}} = \dfrac{180}{60} \)
Simplify the expression:
\( \cot(\theta) = 3 \)
Now, find the angle using the inverse cotangent function:
\( \theta = \cot^{-1}(3) \)
Using a calculator or reference table, the angle is:
∴ θ ≈ 18.4°
4. A photographer is setting up to take a picture of a tall building. The distance from the photographer to the building is 50 meters, and the height of the building is 150 meters. What is the angle of elevation of the camera to the top of the building?
Answer
Given:
- Length of the adjacent side (distance from the building) = 50 meters
- Length of the opposite side (height of the building) = 150 meters
First, calculate the cotangent of the angle:
\( \cot(\theta) = \dfrac{\text{distance from the building}}{\text{height of the building}} = \dfrac{50}{150} \)
Simplify the expression:
\( \cot(\theta) \approx 0.333 \)
Now, find the angle using the inverse cotangent function:
\( \theta = \cot^{-1}(0.333) \)
Using a calculator or reference table, the angle is:
∴ θ ≈ 71.6°