sec Calculator
θ angle
sec(θ)
Calculating Secant of an Angle
The secant (sec) of an angle in a right-angled triangle is a trigonometric function that represents the ratio of the length of the hypotenuse to the adjacent side. The secant function is the reciprocal of the cosine function and is often used in various trigonometric calculations.
The secant of an angle \( \theta \) is defined as:
\( \sec(\theta) = \dfrac{\text{length of hypotenuse}}{\text{length of adjacent side}} \)
where:
- hypotenuse is the side opposite the right angle.
- adjacent side is the side next to the angle \( \theta \), excluding the hypotenuse.
Consider the following triangle ABC, right-angled at vertex B.
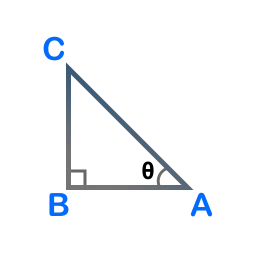
For the angle \( \theta \) at vertex A:
- Hypotenuse = AC
- Adjacent side = AB
Therefore,
\( \sec(\theta) = \dfrac{\text{length of side AC}}{\text{length of side AB}} \)
Examples
The following examples demonstrate how to use the secant of an angle to find the length of the hypotenuse or the adjacent side in various practical situations.
1. What is the secant of an angle θ if the length of the hypotenuse is 10 units and the length of the adjacent side is 8 units?
Answer
Given:
- Length of the hypotenuse = 10 units
- Length of the adjacent side = 8 units
The formula to calculate the secant of an angle θ is:
\( \sec(\theta) = \dfrac{\text{length of hypotenuse}}{\text{length of adjacent side}} \)
Substitute the given values into the formula:
\( \sec(\theta) = \dfrac{10}{8} \)
Simplify the expression:
\( \sec(\theta) = 1.25 \)
∴ sec(θ) = 1.25
2. A person is walking along a path that rises at an angle of 30° to the horizontal. If the horizontal distance covered is 50 meters, what is the length of the path?
Answer
Given:
- Horizontal distance (adjacent side) = 50 meters
- Angle with the horizontal θ = 30°
Using the secant function to find the length of the path (hypotenuse):
\( \sec(30°) = \dfrac{\text{length of path}}{50} \)
Simplify the expression:
\( 1.154 = \dfrac{\text{length of path}}{50} \)
Now solve for the length of the path:
\( \text{Length of path} = 1.154 \times 50 = 57.7 \text{ meters} \)
∴ The length of the path is 57.7 meters.
3. A ladder is placed against a wall at a 60° angle with the ground. If the base of the ladder is 5 meters away from the wall, how long is the ladder?
Answer
Given:
- Distance from the wall (adjacent side) = 5 meters
- Angle with the ground θ = 60°
Using the secant function to find the length of the ladder (hypotenuse):
\( \sec(60°) = \dfrac{\text{length of ladder}}{5} \)
Simplify the expression:
\( 2 = \dfrac{\text{length of ladder}}{5} \)
Now solve for the length of the ladder:
\( \text{Length of ladder} = 2 \times 5 = 10 \text{ meters} \)
∴ The ladder is 10 meters long.