Convert OnlineConvertOnline
  1. Convert Online›
  2. Mathematics›
  3. Trigonometry›
  4. Calculate Sine

sin Calculator

θ angle




sin(θ)

_



Calculating Sin of an Angle

The sine (sin) of an angle in a right-angled triangle is a trigonometric function that represents the ratio of the length of the opposite side to the hypotenuse. The sine function is generally used to find unknown side lengths or angles in right triangles.

The sine of an angle \( \theta \) is defined as:

\( \sin(\theta) = \dfrac{\text{length of opposite side}}{\text{length of hypotenuse}} \)

where:

Consider the following triangle ABC, right angled at vertex B.

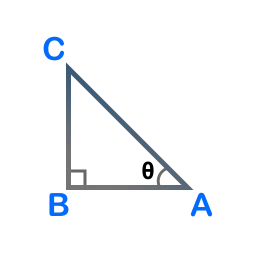
Right Angled Triangle to Calculate sin of angle

For the angle \( \theta \) at vertex A:

Therefore,

\( \sin(\theta) = \dfrac{\text{length of opposite side}}{\text{length of hypotenuse}} \)\( = \dfrac{\text{length of side BC}}{\text{length of side AC}} \)

Examples

The following examples cover how to use the sine of an angle to find the length of the opposite side or the hypotenuse in different practical scenarios.

1. What is the sine of an angle θ if the length of the opposite side is 3 units and the length of the hypotenuse is 5 units?

Answer

Given:

  • Length of opposite side = 3 units
  • Length of hypotenuse = 5 units

The formula to calculate the sine of an angle θ is:

\( \sin(\theta) = \dfrac{\text{length of opposite side}}{\text{length of hypotenuse}} \)

Substitute the given values into the formula:

\( \sin(\theta) = \dfrac{3}{5} \)

Simplify the expression:

\( \sin(\theta) = 0.6 \)

∴ sin(θ) = 0.6

2. A person is flying a kite at an angle of 45° to the ground. If the string of the kite (hypotenuse) is 100 meters long, how high is the kite above the ground?

Answer

Given:

  • Length of the string (hypotenuse) = 100 meters
  • Angle with the ground θ = 45°

Using the sine function to find the height (opposite side) of the kite:

\( \sin(45°) = \dfrac{\text{height}}{100} \)

Simplify the expression:

\( 0.707 = \dfrac{\text{height}}{100} \)

Now solve for the height:

\( \text{Height} = 0.707 \times 100 = 70.7 \text{ meters} \)

∴ The kite is 70.7 meters above the ground.

3. A ladder is leaning against a wall, making a 60° angle with the ground. If the height from the ground to the top of the wall is 8 meters, how long is the ladder?

Answer

Given:

  • Height of the wall (opposite side) = 8 meters
  • Angle of the ladder with the ground θ = 60°

Using the sine function to find the length of the ladder (hypotenuse):

\( \sin(60°) = \dfrac{8}{\text{length of ladder}} \)

Simplify the expression:

\( 0.866 = \dfrac{8}{\text{length of ladder}} \)

Now solve for the length of the ladder:

\( \text{Length of ladder} = \dfrac{8}{0.866} \approx 9.24 \text{ meters} \)

∴ The ladder is approximately 9.24 meters long.

4. A cable is attached to the top of a 50-meter-high tower and secured to the ground at a 30° angle. What is the length of the cable?

Answer

Given:

  • Height of the tower (opposite side) = 50 meters
  • Angle with the ground θ = 30°

Using the sine function to find the length of the cable (hypotenuse):

\( \sin(30°) = \dfrac{50}{\text{length of cable}} \)

Simplify the expression:

\( 0.5 = \dfrac{50}{\text{length of cable}} \)

Now solve for the length of the cable:

\( \text{Length of cable} = \dfrac{50}{0.5} = 100 \text{ meters} \)

∴ The length of the cable is 100 meters.